A Change In Which Of The Following Is Unlikely To Disrupt The Learningã¢â‚¬â€¹ Curve?

Chapter sixteen. Thermodynamics

sixteen.two Entropy

Learning Objectives

By the end of this section, you volition be able to:

• Define entropy
• Explain the relationship between entropy and the number of microstates
• Predict the sign of the entropy change for chemic and concrete processes

In 1824, at the age of 28, Nicolas Léonard Sadi Carnot (Figure 1) published the results of an extensive report regarding the efficiency of steam heat engines. In a later review of Carnot's findings, Rudolf Clausius introduced a new thermodynamic property that relates the spontaneous heat flow accompanying a process to the temperature at which the procedure takes place. This new property was expressed equally the ratio of the reversible estrus (q _rev) and the kelvin temperature (T). The term reversible process refers to a process that takes identify at such a ho-hum rate that information technology is always at equilibrium and its direction can exist inverse (it can be "reversed") by an infinitesimally minor change is some condition. Annotation that the idea of a reversible procedure is a formalism required to support the development of various thermodynamic concepts; no existent processes are truly reversible, rather they are classified every bit irreversible.

Figure one. (a) Nicholas Léonard Sadi Carnot'southward enquiry into steam-powered machinery and (b) Rudolf Clausius'due south later study of those findings led to groundbreaking discoveries almost spontaneous heat flow processes.

Similar to other thermodynamic properties, this new quantity is a country function, so its alter depends only upon the initial and final states of a arrangement. In 1865, Clausius named this property entropy (South) and defined its alter for whatever process as the following:

[latex]{\Delta}South = \frac{q_{\text{rev}}}{T}[/latex]

The entropy change for a real, irreversible process is and so equal to that for the theoretical reversible process that involves the same initial and final states.

Entropy and Microstates

Following the work of Carnot and Clausius, Ludwig Boltzmann developed a molecular-scale statistical model that related the entropy of a organization to the number of microstates possible for the organization. A microstate (W) is a specific configuration of the locations and energies of the atoms or molecules that comprise a organisation like the following:

[latex]South = g\;\text{ln}\;West[/latex]

Hither yard is the Boltzmann constant and has a value of 1.38 × 10⁻²³ J/K.

As for other land functions, the change in entropy for a procedure is the divergence between its final (South _f) and initial (S _i) values:

[latex]{\Delta}S = S_{\text{f}}\;-\;S_{\text{i}} = k\;\text{ln}\;W_{\text{f}}\;-\;grand\;\text{ln}\;W_{\text{i}} = thousand\;\text{ln}\;\frac{W_{\text{f}}}{W_{\text{i}}}[/latex]

For processes involving an increase in the number of microstates, W _f > Westward _i, the entropy of the system increases, ΔS > 0. Conversely, processes that reduce the number of microstates, Due west _f < Westward _i, yield a decrease in system entropy, ΔSouthward < 0. This molecular-scale interpretation of entropy provides a link to the probability that a procedure will occur as illustrated in the adjacent paragraphs.

Consider the general example of a organisation comprised of N particles distributed amidst northward boxes. The number of microstates possible for such a system is n^N . For example, distributing four particles among two boxes volition result in ii^four = sixteen different microstates as illustrated in Figure ii. Microstates with equivalent particle arrangements (not considering private particle identities) are grouped together and are called distributions. The probability that a system will be with its components in a given distribution is proportional to the number of microstates within the distribution. Since entropy increases logarithmically with the number of microstates, the most probable distribution is therefore the one of greatest entropy.

Figure 2. The 16 microstates associated with placing four particles in ii boxes are shown. The microstates are collected into five distributions—(a), (b), (c), (d), and (eastward)—based on the numbers of particles in each box.

For this system, the most probable configuration is one of the half dozen microstates associated with distribution (c) where the particles are evenly distributed between the boxes, that is, a configuration of ii particles in each box. The probability of finding the system in this configuration is or [latex]\frac{vi}{xvi}[/latex] or [latex]\frac{3}{8}[/latex]. The least probable configuration of the system is one in which all four particles are in one box, corresponding to distributions (a) and (d), each with a probability of [latex]\frac{1}{16}[/latex]. The probability of finding all particles in just one box (either the left box or right box) is then [latex](\frac{1}{xvi}\;+\;\frac{i}{16}) = \frac{2}{sixteen}[/latex] or [latex]\frac{1}{eight}[/latex].

Equally y'all add together more particles to the organization, the number of possible microstates increases exponentially (2 ^Northward ). A macroscopic (laboratory-sized) organisation would typically consist of moles of particles (N ~ x²³), and the corresponding number of microstates would be staggeringly huge. Regardless of the number of particles in the system, even so, the distributions in which roughly equal numbers of particles are found in each box are always the most likely configurations.

The previous clarification of an ideal gas expanding into a vacuum (Chapter sixteen.1 Spontaneity) is a macroscopic case of this particle-in-a-box model. For this system, the most likely distribution is confirmed to be the i in which the matter is most uniformly dispersed or distributed between the ii flasks. The spontaneous process whereby the gas independent initially in ane flask expands to fill both flasks equally therefore yields an increase in entropy for the organization.

A like approach may be used to describe the spontaneous flow of estrus. Consider a system consisting of two objects, each containing two particles, and two units of energy (represented equally "*") in Figure 3. The hot object is comprised of particles A and B and initially contains both free energy units. The cold object is comprised of particles C and D, which initially has no energy units. Distribution (a) shows the three microstates possible for the initial state of the system, with both units of free energy contained within the hot object. If one of the two energy units is transferred, the result is distribution (b) consisting of iv microstates. If both energy units are transferred, the result is distribution (c) consisting of three microstates. And so, we may describe this arrangement by a total of ten microstates. The probability that the heat does non menstruum when the two objects are brought into contact, that is, that the system remains in distribution (a), is [latex]\frac{iii}{x}[/latex]. More likely is the flow of heat to yield i of the other 2 distribution, the combined probability being [latex]\frac{7}{ten}[/latex]. The most likely upshot is the menses of heat to yield the compatible dispersal of energy represented past distribution (b), the probability of this configuration being [latex]\frac{4}{10}[/latex]. As for the previous example of matter dispersal, extrapolating this treatment to macroscopic collections of particles dramatically increases the probability of the uniform distribution relative to the other distributions. This supports the mutual observation that placing hot and cold objects in contact results in spontaneous rut menstruum that ultimately equalizes the objects' temperatures. And, again, this spontaneous process is also characterized by an increment in system entropy.

Figure iii. This shows a microstate model describing the menstruation of rut from a hot object to a common cold object. (a) Before the estrus menses occurs, the object comprised of particles A and B contains both units of energy and as represented by a distribution of three microstates. (b) If the heat flow results in an even dispersal of energy (one energy unit transferred), a distribution of 4 microstates results. (c) If both free energy units are transferred, the resulting distribution has three microstates.

Example 1

Decision of ΔS
Consider the system shown here. What is the change in entropy for a procedure that converts the organization from distribution (a) to (c)?

Solution
We are interested in the post-obit modify:

The initial number of microstates is ane, the concluding six:

[latex]{\Delta}S = k\;\text{ln}\;\frac{W_{\text{c}}}{W_{\text{a}}} = ane.38\;\times\;10^{-23}\;\text{J}/\text{K}\;\times\;\text{ln}\;\frac{6}{1} = 2.47\;\times\;10^{-23}\;\text{J}/\text{K}[/latex]

The sign of this consequence is consistent with expectation; since there are more microstates possible for the final state than for the initial state, the change in entropy should exist positive.

Check Your Learning
Consider the organisation shown in Figure 3. What is the alter in entropy for the process where all the energy is transferred from the hot object (AB) to the cold object (CD)?

Predicting the Sign of ΔS

The relationships between entropy, microstates, and matter/energy dispersal described previously allow the states to make generalizations regarding the relative entropies of substances and to predict the sign of entropy changes for chemical and physical processes. Consider the stage changes illustrated in Figure 4. In the solid phase, the atoms or molecules are restricted to nearly stock-still positions with respect to each other and are capable of only small-scale oscillations about these positions. With essentially stock-still locations for the system's component particles, the number of microstates is relatively small. In the liquid phase, the atoms or molecules are free to move over and effectually each other, though they remain in relatively close proximity to one another. This increased freedom of motility results in a greater variation in possible particle locations, so the number of microstates is correspondingly greater than for the solid. Every bit a result, S _liquid > S _solid and the process of converting a substance from solid to liquid (melting) is characterized past an increment in entropy, ΔS > 0. By the same logic, the reciprocal process (freezing) exhibits a decrease in entropy, ΔS < 0.

Effigy iv. The entropy of a substance increases (ΔS > 0) equally it transforms from a relatively ordered solid, to a less-ordered liquid, and then to a nonetheless less-ordered gas. The entropy decreases (ΔS < 0) as the substance transforms from a gas to a liquid so to a solid.

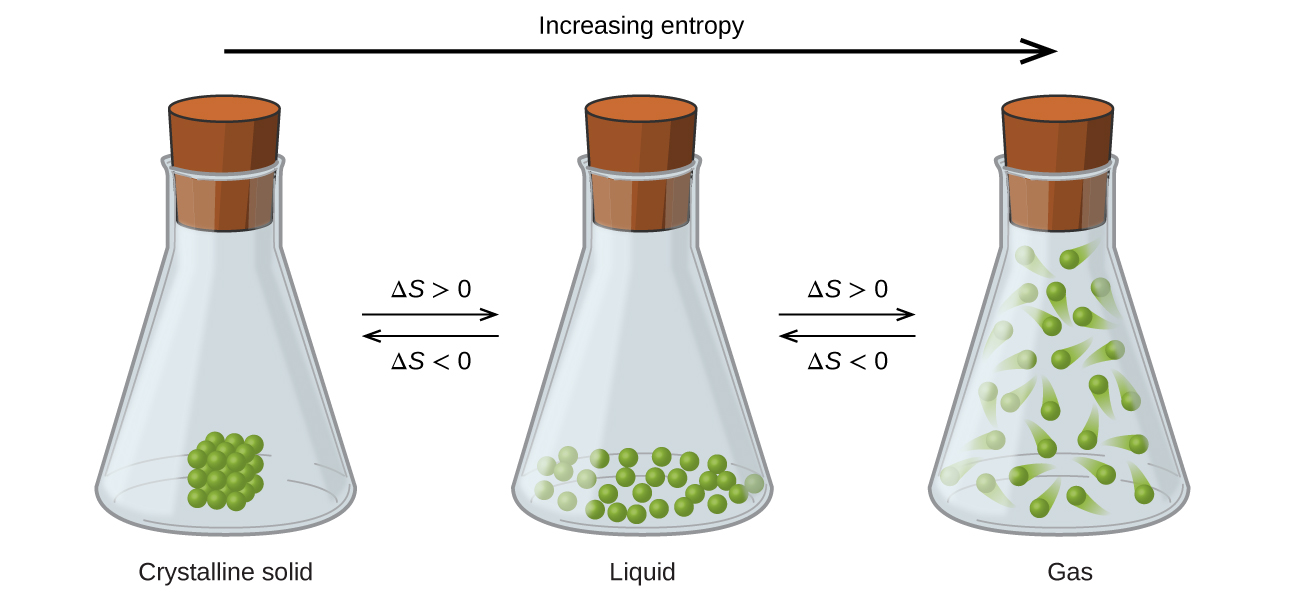
Now consider the vapor or gas phase. The atoms or molecules occupy a much greater book than in the liquid phase; therefore each atom or molecule can be found in many more locations than in the liquid (or solid) phase. Consequently, for whatsoever substance, South _gas > S _liquid > South _solid, and the processes of vaporization and sublimation likewise involve increases in entropy, ΔS > 0. Likewise, the reciprocal phase transitions, condensation and deposition, involve decreases in entropy, ΔDue south < 0.

Co-ordinate to kinetic-molecular theory, the temperature of a substance is proportional to the average kinetic free energy of its particles. Raising the temperature of a substance will event in more all-encompassing vibrations of the particles in solids and more rapid translations of the particles in liquids and gases. At higher temperatures, the distribution of kinetic energies amidst the atoms or molecules of the substance is also broader (more dispersed) than at lower temperatures. Thus, the entropy for whatsoever substance increases with temperature (Figure 5).

Effigy five. Entropy increases as the temperature of a substance is raised, which corresponds to the greater spread of kinetic energies. When a substance melts or vaporizes, it experiences a meaning increment in entropy.

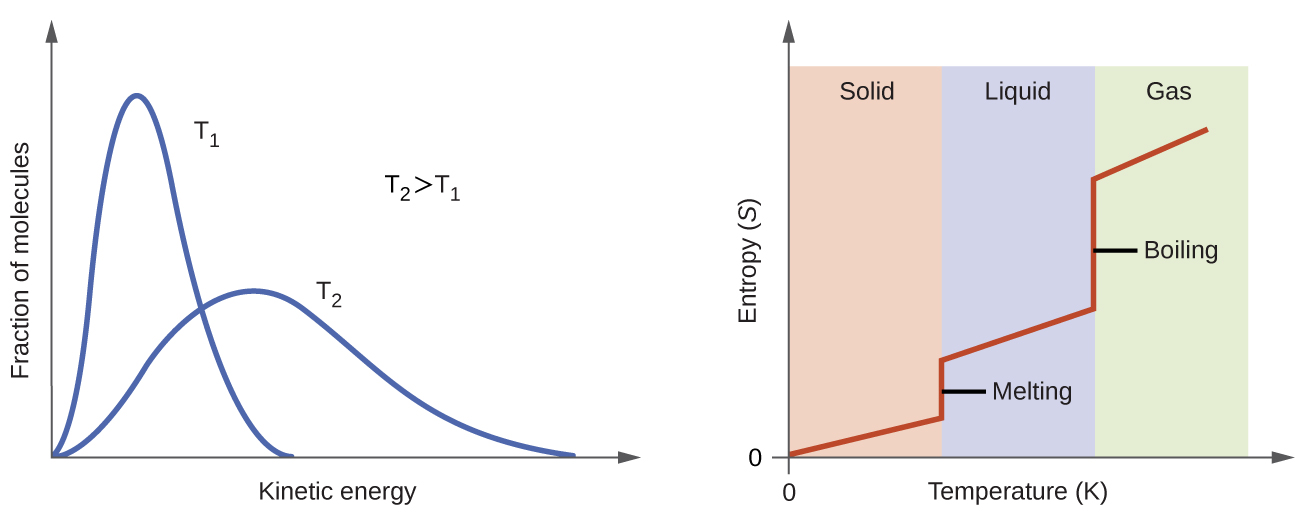
Try this simulator with interactive visualization of the dependence of particle location and freedom of movement on physical state and temperature.

The entropy of a substance is influenced by structure of the particles (atoms or molecules) that comprise the substance. With regard to diminutive substances, heavier atoms possess greater entropy at a given temperature than lighter atoms, which is a consequence of the relation between a particle's mass and the spacing of quantized translational free energy levels (which is a topic beyond the scope of our treatment). For molecules, greater numbers of atoms (regardless of their masses) increment the ways in which the molecules can vibrate and thus the number of possible microstates and the system entropy.

Finally, variations in the types of particles affects the entropy of a arrangement. Compared to a pure substance, in which all particles are identical, the entropy of a mixture of ii or more different particle types is greater. This is because of the boosted orientations and interactions that are possible in a system comprised of nonidentical components. For instance, when a solid dissolves in a liquid, the particles of the solid feel both a greater freedom of movement and additional interactions with the solvent particles. This corresponds to a more uniform dispersal of thing and energy and a greater number of microstates. The process of dissolution therefore involves an increment in entropy, ΔS > 0.

Considering the various factors that impact entropy allows u.s.a. to make informed predictions of the sign of ΔS for various chemical and physical processes as illustrated in Instance 2.

Example 2

Predicting the Sign of ∆South
Predict the sign of the entropy change for the following processes. Indicate the reason for each of your predictions.

(a) Ane mole liquid water at room temperature ⟶⟶ i mole liquid water at 50 °C

(b) [latex]\text{Ag}^{+}(aq)\;+\;\text{Cl}^{-}(aq)\;{\longrightarrow}\;\text{AgCl}(s)[/latex]

(c) [latex]\text{C}_6\text{H}_6(l)\;+\;\frac{fifteen}{2}\text{O}_2(one thousand)\;{\longrightarrow}\;half dozen\text{CO}_2(g)\;+\;3\text{H}_2\text{O}(50)[/latex]

(d) [latex]\text{NH}_3(s)\;{\longrightarrow}\;\text{NH}_3(l)[/latex]

Solution
(a) positive, temperature increases

(b) negative, reduction in the number of ions (particles) in solution, decreased dispersal of matter

(c) negative, net decrease in the corporeality of gaseous species

(d) positive, stage transition from solid to liquid, net increment in dispersal of matter

Check Your Learning
Predict the sign of the enthalpy change for the post-obit processes. Give a reason for your prediction.

(a) [latex]\text{NaNO}_3(s)\;{\longrightarrow}\;\text{Na}^{+}(aq)\;+\;\text{NO}_3^{\;\;-}(aq)[/latex]

(b) the freezing of liquid water

(c) [latex]\text{CO}_2(s)\;{\longrightarrow}\;\text{CO}_2(g)[/latex]

(d) [latex]\text{CaCO}(south)\;{\longrightarrow}\;\text{CaO}(due south)\;+\;\text{CO}_2(m)[/latex]

Respond:

(a) Positive; The solid dissolves to requite an increase of mobile ions in solution. (b) Negative; The liquid becomes a more ordered solid. (c) Positive; The relatively ordered solid becomes a gas. (d) Positive; There is a net production of ane mole of gas.

Key Concepts and Summary

Entropy (Due south) is a state function that tin can be related to the number of microstates for a organisation (the number of means the system can be arranged) and to the ratio of reversible rut to kelvin temperature. It may exist interpreted as a mensurate of the dispersal or distribution of matter and/or energy in a arrangement, and it is oft described equally representing the "disorder" of the arrangement.

For a given substance, S _solid < S _liquid < Due south _gas in a given concrete country at a given temperature, entropy is typically greater for heavier atoms or more complex molecules. Entropy increases when a organisation is heated and when solutions form. Using these guidelines, the sign of entropy changes for some chemical reactions may be reliably predicted.

Key Equations

• [latex]{\Delta}Due south = \frac{q_{\text{rev}}}{T}[/latex]
• [latex]S = thou\;\text{ln}\;W[/latex]
• [latex]{\Delta}S = k\;\text{ln}\;\frac{W_{\text{f}}}{W_{\text{i}}}[/latex]

Chemical science End of Chapter Exercises

1. In Figure 2 all possible distributions and microstates are shown for four dissimilar particles shared between two boxes. Decide the entropy change, ΔS, if the particles are initially evenly distributed between the two boxes, but upon redistribution all end up in Box (b).
2. In Figure 2 all of the possible distributions and microstates are shown for 4 unlike particles shared between ii boxes. Make up one's mind the entropy modify, ΔSouth, for the system when it is converted from distribution (b) to distribution (d).
3. How does the process described in the previous item chronicle to the organisation shown in Chapter 16.ane Spontaneity?
4. Consider a organization like to the 1 in Effigy 2, except that it contains six particles instead of four. What is the probability of having all the particles in only one of the two boxes in the example? Compare this with the similar probability for the organisation of four particles that we take derived to exist equal to [latex]\frac{one}{eight}[/latex]. What does this comparison tell united states nigh even larger systems?
5. Consider the system shown in Figure 3. What is the change in entropy for the process where the energy is initially associated simply with particle A, but in the final country the energy is distributed between two dissimilar particles?
6. Consider the system shown in Figure 3. What is the alter in entropy for the process where the energy is initially associated with particles A and B, and the energy is distributed between ii particles in different boxes (ane in A-B, the other in C-D)?
7. Adapt the following sets of systems in order of increasing entropy. Assume one mole of each substance and the aforementioned temperature for each member of a prepare.

   (a) H₂(chiliad), HBrO₄(g), HBr(g)

   (b) H_iiO(l), H_iiO(g), H₂O(south)

   (c) He(one thousand), Cl₂(chiliad), P₄(g)

8. At room temperature, the entropy of the halogens increases from I₂ to Br₂ to Cl_ii. Explain.
9. Consider two processes: sublimation of I₂(s) and melting of I₂(south) (Note: the latter process tin occur at the aforementioned temperature but somewhat higher pressure).

   [latex]\text{I}_2(s)\;{\longrightarrow}\;\text{I}_2(g)[/latex]

   [latex]\text{I}_2(due south)\;{\longrightarrow}\;\text{I}_2(l)[/latex]

   Is ΔSouthward positive or negative in these processes? In which of the processes will the magnitude of the entropy change be greater?

10. Indicate which substance in the given pairs has the college entropy value. Explain your choices.

    (a) C₂H₅OH(l) or C_threeH_viiOH(l)

    (b) C₂H_vOH(l) or C₂H₅OH(grand)

    (c) 2H(chiliad) or H(thousand)

11. Predict the sign of the entropy change for the following processes.

    (a) An ice cube is warmed to near its melting point.

    (b) Exhaled jiff forms fog on a cold forenoon.

    (c) Snowfall melts.

12. Predict the sign of the entropy modify for the following processes. Give a reason for your prediction.

    (a) [latex]\text{Atomic number 82}^{2+}(aq)\;+\;\text{Southward}^{two-}(aq)\;{\longrightarrow}\;\text{PbS}(s)[/latex]

    (b) [latex]2\text{Atomic number 26}(s)\;+\;iii\text{O}_2(g)\;{\longrightarrow}\;\text{Fe}_2\text{O}_3(s)[/latex]

    (c) [latex]2\text{C}_6\text{H}_{fourteen}(l)\;+\;19\text{O}_2(g)\;{\longrightarrow}\;fourteen\text{H}_2\text{O}(g)\;+\;12\text{CO}_2(g)[/latex]

13. Write the counterbalanced chemical equation for the combustion of marsh gas, CH₄(g), to give carbon dioxide and water vapor. Explain why information technology is hard to predict whether ΔSouth is positive or negative for this chemical reaction.
14. Write the balanced chemical equation for the combustion of benzene, C₆H_six(l), to give carbon dioxide and water vapor. Would yous expect ΔS to exist positive or negative in this process?

Glossary

entropy (S)

state office that is a mensurate of the thing and/or energy dispersal within a system, determined by the number of system microstates ofttimes described as a measure of the disorder of the system

microstate (West)

possible configuration or arrangement of matter and energy inside a system

reversible process

process that takes place so slowly as to be capable of reversing management in response to an infinitesimally minor change in conditions; hypothetical construct that can only be approximated by real processes removed

Solutions

Answers to Chemistry Finish of Chapter Exercises

two. There are four initial microstates and four terminal microstates.
[latex]{\Delta}S = m\;\text{ln}\;\frac{W_{\text{f}}}{W_{\text{i}}} = 1.38\;\times\;x^{-23}\;\text{J}/\text{One thousand}\;\times\;\text{ln}\;\frac{4}{4} = 0[/latex]

4. The probability for all the particles to exist on ane side is [latex]\frac{1}{32}[/latex]. This probability is noticeably lower than the [latex]\frac{1}{viii}[/latex] issue for the 4-particle system. The conclusion nosotros can brand is that the probability for all the particles to stay in only i part of the system will decrease rapidly as the number of particles increases, and, for case, the probability for all molecules of gas to gather in only one side of a room at room temperature and pressure is negligible since the number of gas molecules in the room is very large.

6. There is just one initial state. For the terminal state, the energy can exist contained in pairs A-C, A-D, B-C, or B-D. Thus, there are four last possible states.
[latex]{\Delta}Southward = k\;\text{ln}\;(\frac{W_{\text{f}}}{W_{\text{i}}}) = 1.38\;\times\;ten^{-23}\;\text{J}/\text{Grand}\;\times\;\text{ln}\;(\frac{4}{1}) = 1.91\;\times\;ten^{-23}\;\text{J}/\text{Yard}[/latex]

eight. The masses of these molecules would advise the contrary trend in their entropies. The observed trend is a upshot of the more meaning variation of entropy with a physical land. At room temperature, I₂ is a solid, Br_ii is a liquid, and Cl₂ is a gas.

ten. (a) C₃H₇OH(50) as it is a larger molecule (more than complex and more massive), and then more than microstates describing its motions are bachelor at whatsoever given temperature. (b) C₂H₅OH(g) as it is in the gaseous state. (c) 2H(g), since entropy is an extensive holding, and then two H atoms (or ii moles of H atoms) possess twice as much entropy as one atom (or one mole of atoms).

12. (a) Negative. The relatively ordered solid precipitating decreases the number of mobile ions in solution. (b) Negative. There is a net loss of three moles of gas from reactants to products. (c) Positive. There is a net increase of seven moles of gas from reactants to products.

14. [latex]\text{C}_6\text{H}_6(50)\;+\;7.5\text{O}_2(grand)\;{\longrightarrow}\;3\text{H}_2\text{O}(yard)\;+\;6\text{CO}_2(g)[/latex]
At that place are seven.5 moles of gas initially, and 3 + half-dozen = 9 moles of gas in the terminate. Therefore, it is likely that the entropy increases as a upshot of this reaction, and ΔS is positive.

Source: https://opentextbc.ca/chemistry/chapter/16-2-entropy/

Posted by: sparkmananable72.blogspot.com

Share this post